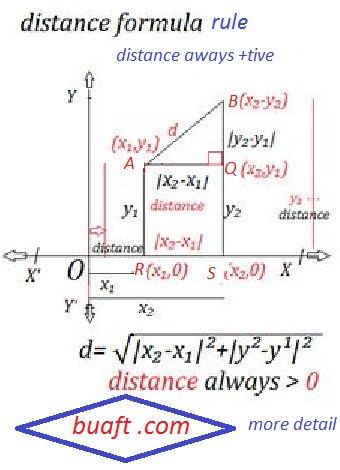
Distance formula rule, formula for the distance d. The distance is always taken to be positive, and it is not a directed distance from A to B. When A and B do not lie on the same horizontal or vertical line.
If A and B lie on a line parallel to one of the coordinate Axis. Then, by the formula 2,The distance AB is the absolute value of the directed distance → AB

Distance formula Proof:
Let A(x ₁, y ₁)and B(x ₂, y ₂) be two point in the plane. We can find distance from the right triangle AQB by using the pathagoras theorem, we have
Distance formula rule
d² = AB² = A Q² + QB² →→→(1)
∣A Q∣ = ∣RS∣ = ∣RO+OS∣
∣RS∣=∣-OR+OS∣
= ∣x ₁-x ₁∣
∣QB∣= ∣SB-SQ∣=∣OM-ON∣
= ∣y ₁-y ₁∣
therefore, (1) can be written in the form
d²= d=(x ₂- x ₁)²+(y ₂- y ₁)²
|
RELATED POST:
- circle of diameter symbol example
- dimensional system
- Circle passing through two point
- Foci of the ellipse, covertices, major and minor axis
- point of inflexion derivative rule
- Finding common logarithm of any number