Unit and unity in ring theory, The term unit and unity should not be confused. Unity is the multiplicative identity, while a unit is any element having a multiplicative inverse. Thus, the multiplicative identity or unity is a unit, but every unit is not a unity. For example, -1 is a unit in the ring (Z, +, ×) of all integers, but -1 is not a unity, that is -1 ≠ 1
Commutative ring definition:
A ring in which multiplication is commutative is called a commutative ring.
Unity in ring:
A ring (R, +, ×) with multiplicative identity 1 such that 1×a=a×1 =a for all a ∈ R is called a ring with Unity. The multiplicative identity in a ring is called a unity.
Unity ring Explanation: unit and unity ring theory
In a ring with unity, the non-zero elements satisfy all the axioms for a group under the multiplication, except possibly the existence of multiplicative inverse. A multiplicative inverse of an element a. in a ring(R, +, ×) with unity 1 is an element a ‾ ¹ ∈ R such that a × a ‾ ¹ = a ‾ ¹× a = 1 Since 0 ×a = a × 0 cannot have a multiplicative inverse unless we regard the set {0}, where 0 + 0 = 0 and (0)×(0) = 0 as a ring with zero as both the additive and multiplicative identity. We agree to exclude this travel case whenever we speak of a ring with unity, that is, we shall regard a ring with unity to be a nonzero ring.
Division ring:
Let (R, +, ×) be a ring with unity. An element a ∈ R is called a unit of (R, +, ×). If it has a multiplicative inverse in R. if every nonzero element of R is unit, Then(R, +, ×) is called a division ring or skew field.
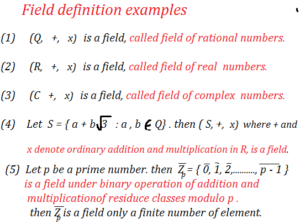
Field:
A commutative division ring is called a field.
Field definition:
A field (F, +, ×) is a non-empty set F having at least two elements and two binary operation + and × (addition and multiplication) defined on F such that the following Axioms are satisfied.
(1) (F, +) is an Abelian group under addition.
(2) (F – {0}, ×} is an Abelian group under multiplication.
(3) For all a, b, c ∈ F, the right distributive law holds:
that is
(a + b) c = ac + bc