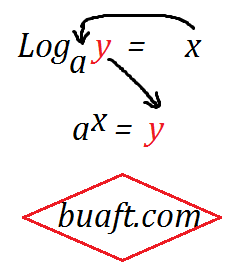Log Rule Exponential Of Real Number

Log rule exponential:

then x is called the logarithm of y to base a

and written as

$\log _{a}y=x$

where a >0, a ≠ 1 and y >0

That is

The logarithms of a number y to the base’ a’ is the index of x

of the power to which a must be raised to get that number y.

The relation

and $\log _{a}y=x$ are equivalent.

When one relation is given, it can be converted into the other.

Thus log rule exponential

⇔ $\log _{a}y=x$

and $\log _{a}y=x$ are respectively exponential and logarithms form

of the same solution

Logarithms example: 1

$log rule exponential$

Logarithms example: 2

$log rule exponential$

Conversely, rule loge

$\log _{3}27=3\, \, is\, equivalent \, \, to 27= 3^{3}$

Logarithm of a negative number is not fined at this stage.

Logarithm:

Logarithms are useful tools for accurate and rapid computations

Logarithms base 10

Logarithms base 10 are known as common logarithms

Natural logarithms

Logarithms with base e are called natural logarithms

log rule exponential of real number