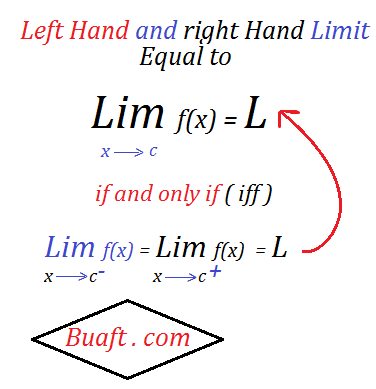
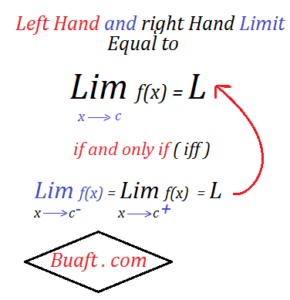
Left-hand limit and right-hand limit:
Left-hand limit:
The limit of f(x) is equal to L As x approaches c from the left, That is for all x sufficiently close to c, but less than c, The value of f(x) can be made as close we please to L.
x approaches to infinity means
Left-hand limit symbolic form:
Right-hand limit:
The Limit of the f(x) is equal to M as x approaches to c from the right, that is for all x sufficiently close to c, the value of f(x) can be made as close we please to M.
Right-hand limit symbolic form:
Rule of left-hand limit and right-hand limit:
The rule of calculating the Negative-hand Limit and positive-hand Limit are the same
One-sided Limit:
In defining , We restricted x to an open interval containing c that is we studied the behavior of f on both side of c. However, in some cases it is necessary to investigate one-sided limit, Negative-hand Limit and Positive-hand Limit. We have already described.
RELATED POST
- Limit of a function concept
- ♣
- Cube root of unity power
- ♣
- Fourth roots of unity power
- ♣
- branches of curve of hyperbola
- ♣
- radicals and radicands
- ♣
- Addition and multiplication laws of real number
- ♣
- commutative property of rational number