Associative property is explained as, more as two numbers are added (+) or multiplied(x), give the result remains as same, For example we have three real numbers a, b. c
a +(b+c) = a +(b+c) condition one
a ×(b×c) = a ×(b×c) condition two
All numbers natural:
N ={1,2,3,4………………∞(…:mean all natural numbers without undefined
whole number:
W= {0,1,2,3,4,5,6………….∞(
set of integer: Z=)∞……..-6,-5,-4,-3,-2,-1,0,+1,+2,+3,+4,+5,+6……..∞(
Rational number :
Q={-1….-.5….. 0…1.5….1…1.5…..2….}
Irrational number Q’ ={all numbers which contain non-terminating and non-recurring decimal or numbering result is called irrational number}
Real number R = {set of all number N+W+Z+Q+Q’ called real number set}
We will discuss the associative property of real number addition, multiplication, negation and division.
Any number a, b, c we check these properties verify or not:
(1) associative property of addition
(2) associative property of multiplication
(3) associative property of subtraction
(4) associative property of division
associative property mean number objects and thing in the world we verify that
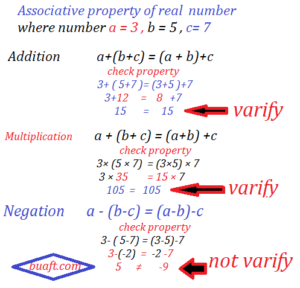
(1) associative property of addition:
(a+b)+c= a+(b+c) …………………….. a
we take a numeric number 4, 6, 9 and apply on association
(4+6)+9= 4+(6+9)
10+9= 4+15
19=19
these number are verified associative property of real number.
Hence, addition of associative property verify
(2) associative property of multiplication:
now we take any three real numeric 1, 1/2, 2/4and check multiplication on associative property
(a+b)+c= a+(b+c)
(1+1/2)+2/4= 1+(1/2+2/4) by algebraic operation
3/2+1/2= 1+1
2 = 2
or
1 = 1
hence, verify association on these numbers
therefore, associative property is verifying the multiplication
(3) associative property of negation:
we take a three real number a=5, b= 6, c=7
associative property of negation on any number
a-(b-c)=(a-b)-c……….(a)
putting the value of a, b, c in association property “a”
5-(6-7)=(5-6)-7
5+1= -1-7
6= -6 this is not equal
association property of negative value of real number not hold
(4) associative property of division

we take any three real number a= 2, b= 8,c=12
(a÷b)÷c= a÷(b÷c)……….(B)
putting the value of real number
(2÷8)÷12= 2÷(8÷12)
1/4÷12 =2÷2/3
by algebraic rule apply
1/4×1/12 =2×1/12
1/48 = 1/6………..not equal
hence, the property of association of division is not applicable
RELATED POST
- type of set
- ♥
- relation concept example
- ♥
- equal set writing method
- ♣
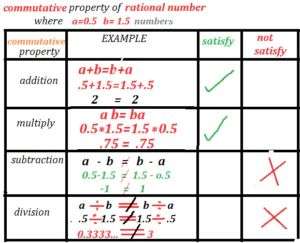
- commutative property of rational number
- ♣
- properties of determinant proves
- ♦
- Foci of the ellipse, covertices, major and minor axis