properties of binary operation:Suppose that S be a non empty set And ∗ (read star) be a binary operation on set S ( means set S is closed with rest to ∗ ) Then ∗ may verify the following properties.
Suppose set S = { 1,7, 9} On this set we apply the following properties
a = 1 , b = 7 , c = 9
- Commutative property
If for all a, b ∈ S (∀ a, b is a part of S)
this implies that ( ⇒ )
a ∗ b = b ∗ a
Then ∗ is commutative in S.
EXAMPLE:
1 ∗ 7 = 7∗ 1
7 = 7
Hence verified.
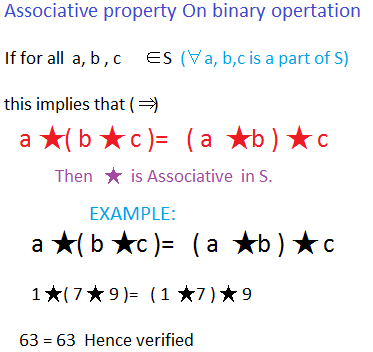
- Associative property
If for all a, b , c ∈ S (∀ a, b,c is a part of S)
this implies that ( ⇒ )
a ∗ ( b ∗ c )= ( a ∗ b ) ∗ c
Then ∗ is Associative in S.
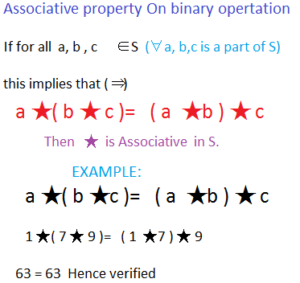
EXAMPLE:
a ∗ ( b ∗ c )= ( a ∗ b ) ∗ c
1 ∗ ( 7 ∗ 9 )= ( 1 ∗ 7 ) ∗ 9
63 = 63
Hence verified.
- Identity property
If for all a ∈ S (∀ a is a part of S)
there exist e ∈ S ( e is a part of S)
Such that
a ∗ e = e ∗ a
a = a
Then e is called identity w . r . t ∗ ( binary operation )
EXAMPLE:
a ∗ e = e ∗ a
1 ∗ 1 = 1 ∗ 1
1 = 1
Hence verified.
- Inverse property
If for all a ∈ S (∀ a is a part of S)
there exist a’ ∈ S ( inverse of a is a part of set S)
such that
a ∗ a’= a’ ∗ a
e = e
Then a’ ( inverse of a ) is called inverse of a with respect to
∗ ( binary operation).
EXAMPLE:
7 ∗ 1/7 = 1/7 ∗ 7
1 = 1
1 = 1
Hence verified.
RELATED POST: