Term Monoid in set theory

Term Monoid in set theory; A semi group is Monoid  If it has identity element with respect to binary operation  ∗  .Term Monoid in set theory image.

A non empty set G is said to be monoid if the following condition verify

1.  G is closed w . r . t    binary operation   ∗
2.   G is associative  w . r . t   binary operation     ∗
3.   G  has an identity element w . r . t binary operation   ∗

Term Monoid in set theory;

Example : 

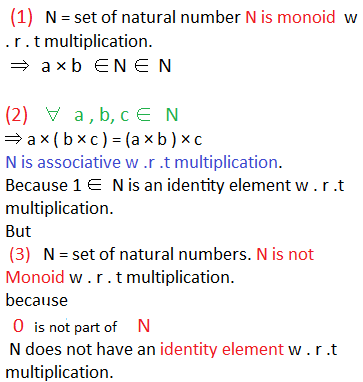
N = set of natural number N is monoid  w . r . t multiplication.

because ∀ ( for all ) a , b ∈ N  (N =1, 2 ,3,4…………).

This implies that a × b  ∈ N ∈ N ( ∈ means is a part of N).

∀ ( for all ) a , b, c ∈ N  (N =1, 2 ,3,4…………).

(This implies that ) ⇒   a × ( b × c ) = (a × b ) × c

Shows that N is associative w .r .t multiplication.

Because 1 ∈ N is an identity element w . r .t multiplication.

But

N = set of natural numbers. N is not Monoid w . r . t multiplication.

because   0 ∉ N ( zero is not a part of natural number).

So N does not have an identity element w . r .t multiplication.

Term Group in set theory :

A monoid is said to be a  group if  its each element have inverse w . r .t    ∗

or

A non empty set G is said to be a group w . r .t binary operation ∗

1.  G is closed w . r . t    binary operation   ∗
2.   G is associative  w . r . t   binary operation     ∗
3.   G  has an identity element w . r . t binary operation   ∗
4. G  has inverse of each element w . r .t binary operation   ∗

Term group Example:

Let  ℝ – { 0 } be the set of non zero real numbers.

Then

ℝ – { 0 } is a group w . r . t  multiplication

Because

1. ℝ – { 0 }  is closed w . r . t    binary operation         multiplication.
2.   ℝ – { 0 } is associative  w . r . t   binary operation multiplication.
3. ℝ – { 0 }   has an identity element w . r . t binary operation multiplication.

ℝ – { 0 }  has inverse of each element w . r .t binary operation  multiplication.

RELATED POST:
properties of binary operation

Cartesian product of two set

singleton set has proper subset